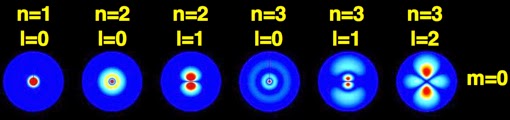
Find $N$ (normalized) wave functions $\psi_1(x,t),\psi_2(x,t),...,\psi_N(x,t)$ depending on a 3d space coordinate $x$ and time coordinate $t$ with non-overlapping spatial supports $\Omega_1(t),\Omega_2(t),...\Omega_N(t)$ filling 3d space, such that the combined wave function $\psi (x,t)$ defined by $\psi (x,t)=\psi_j(x,t)$ for $x\in\Omega_j(t)$ for $j=1,...,N,$ satisfies
- $\ddot\psi (x,t)+H^2\psi(x,t) =0$ for all $(x,t)$, (1)
where the (normalized) Hamiltonian $H=H(x)$ is given by
- $H(x) = -\frac{1}{2}\Delta - \frac{N}{\vert x\vert}+\sum_{k\neq j}\int\frac{\psi_k^2(y,t)}{2\vert x-y\vert}dy$, for $x\in\Omega_j(t)$.
The combined wave function $\psi (x,t)$ is thus assumed to be continuous, more precisely so smooth that $H^2\psi (x,t)$ is defined, and the potential acting in $\Omega_j(t)$ is given as the attractive kernel potential together with the repulsive kernel potential resulting from the combined electronic charge distributions $\psi_k^2$ for $k\neq j$.
The time-independent ground state $\psi (x)$ satisfying $H\psi =E\psi$ with minimal energy $E$ is then determined as the minimizer of the total energy functional
The time-independent ground state $\psi (x)$ satisfying $H\psi =E\psi$ with minimal energy $E$ is then determined as the minimizer of the total energy functional
- $\frac{1}{2}\int\vert\nabla\psi\vert^2 dx-\int\frac{N\psi^2}{\vert x\vert}dx+\sum_{k\neq j}\int_{\Omega_j}\int_{\Omega_k}\frac{\psi_j^2(x_j)\psi_k^2(x_k)}{2\vert x_j-x_k\vert}dx_jdx_k$.
This is a free-boundary electron (or charge) density formulation keeping the individuality of the electrons, which can be viewed as a "smoothed $N$-particle problem" of interacting non-overlapping "electron clouds" under Laplacian smoothing. The model (1) connects to the study in Quantum Contradictions showing a surprisingly good agreement with observations.
For example, the ground state of Helium in this model consists of two disjoint (contacting) half-spherical electron clouds with energy in close agreement with observation, see Quantum Contradictions 12, and PS2 below.
This is to be compared with the postulated ground state according to the standard Schrödinger equation considered to be two overlaying spherical electron configurations named as $1s2$, which however has wrong energy and thus is not the true ground state, and thus asks for perturbative correction.
PS1 In the series Quantum Contradictions 1-29 we considered a model closely related to (1) with each one-electron wave function $\psi_j$ a smooth function defined in 3d space satisfying a one-electron wave equation with the Laplacian smoothing acting on the sum of the wave functions, and not on each individual wave function, with the effect of reducing contributions to the smoothing energy from angular variation. In this case the one-electron wave functions have overlapping support, while being largely separated by repulsion and loosing individuality under overlapping Laplacian smoothing.
PS2 Computing an approximation of the ground-state energy $E$ of Helium using the two normalized separated half-spherical wave functions
- $\psi_1(x)=\sqrt{\frac{2\alpha^3}{\pi}}\exp(-\alpha\vert x\vert )$ for $x=(x_1,x_2,x_3)$ with $x_3\ge 0$
- $\psi_2(x)=\sqrt{\frac{2\alpha^3}{\pi}}\exp(-\alpha\vert x\vert )$ for $x$ with $x_3\le 0$,
- $E=-2.902\pm 0.002$,
to be compared with the observed $-2.903$. The standard 1s2 ground state of two overlaying fully spherical wave functions gives a best value of -2.85 asking for so called perturbation correction, which effectively introduces electron separation. We have thus found evidence that the ground state of Helium is not the standard 1s2 state with two overlaying spherical wave functions, without or with perturbation correction, but instead consists of two non-overlapping half-spherical wave functions. If this conclusion indeed shows to be correct, then electronic wave functions will have to be recomputed for all atoms. Mind-boggling!
PS3 For Li+ we get E = -7.32 (-7.28), for Be2+ we get = -13.72 (-13.65) and for B3+ we get -22.12 (-22.03) with measured value in parenthesis....more to come...
PS3 For Li+ we get E = -7.32 (-7.28), for Be2+ we get = -13.72 (-13.65) and for B3+ we get -22.12 (-22.03) with measured value in parenthesis....more to come...